Difference between revisions of "DCOM Volume I Appendix D"
| Line 48: | Line 48: | ||
* When the slab is rigidly connected to the walls (as is generally the case) the bending moments at the junction between the walls and slab should be taken into account in the design of slab together with any direct forces transferred to the slab from the walls or from the slab to the wall due to suspension of the slab from the wall. | * When the slab is rigidly connected to the walls (as is generally the case) the bending moments at the junction between the walls and slab should be taken into account in the design of slab together with any direct forces transferred to the slab from the walls or from the slab to the wall due to suspension of the slab from the wall. | ||
* If the walls are non-monolithic with the slab, such as in cases, where movement joints have been provided between the slabs and walls, the slab should be designed only for the vertical loads. | * If the walls are non-monolithic with the slab, such as in cases, where movement joints have been provided between the slabs and walls, the slab should be designed only for the vertical loads. | ||
| − | * In continuous T-beams and L-beams with ribs on the side remote from the liquid, the tension in concrete on the liquid side at the face of the supports should not exceed the permissible stresses for controlling cracks in concrete. The width of the slab is determined in usual manner for calculation of the resistance to cracking of T-beam, L-beam sections at supports as given in | + | * In continuous T-beams and L-beams with ribs on the side remote from the liquid, the tension in concrete on the liquid side at the face of the supports should not exceed the permissible stresses for controlling cracks in concrete. The width of the slab is determined in usual manner for calculation of the resistance to cracking of T-beam, L-beam sections at supports as given in BS8110 design code. |
| − | BS8110 design code. | ||
'''Circular Tanks with sliding joint at base:'''<br> | '''Circular Tanks with sliding joint at base:'''<br> | ||
| − | * If the wall of a cylindrical tank has a sliding joint at the base and is free at the | + | * If the wall of a cylindrical tank has a sliding joint at the base and is free at the top, then when the tank is full, no radial shear or vertical bending occurs, the tank wall will be subjected to pure circumferential tension with a varying |
| − | top, then when the tank is full, no radial shear or vertical bending occurs, | + | magnitude whereby at bottom there is maximum value and at top there is zero value, |
| − | the tank wall will be subjected to pure circumferential tension with a varying | ||
| − | magnitude whereby at bottom there is maximum value and at top there is | ||
| − | zero value, | ||
* The design of the tank wall should be done by determining the width of the tank wall t and the area of reinforcement Ast required to resist the circumferential tension only, | * The design of the tank wall should be done by determining the width of the tank wall t and the area of reinforcement Ast required to resist the circumferential tension only, | ||
| + | * The varying value of circumferential tension per unit height T at depth z below the top and area of steel required to resist circumferential tension and thickness of wall are given by equations below: | ||
| + | |||
| + | [[Image:AppendixDfomula.PNG|159px|link=DCOM_Volume_I]] | ||
| + | Where: | ||
| + | r is the internal radius of the tank , | ||
| + | γ is unit weight of the liquid, | ||
| + | z is the depth below the top of tank, | ||
| + | Ast is the area of circumferential tension steel, | ||
| + | σst is the permissible tension strength of steel, | ||
| + | T is circumferential tension (Hoops tension), | ||
| + | |||
| + | '''Circular Tank with fixed joint at base:'''<br> | ||
| + | * If the wall of the Tank is supported at the base such that no radial movement occurs; then the wall will be subjected to radial shear, vertical bending and circumferential tension, the value of circumferential tension is always zero at the bottom of the wall, | ||
| + | * The assumption should be made that some portion of the wall at base acts as cantilever and thus some load at bottom are taken by the cantilever effect. Load in the top portion is taken by the hoop tension. The cantilever effect depends on the height of the wall, | ||
| + | * The bottom part of the tank wall about 13 of the tank height H, or 1 meter from bottom whichever is greater is acted upon by a cantilever moment, | ||
| + | * For walls with free tops and a bottom that is either fixed or hinged values of circumferential tension, vertical moments and radial shear may be calculated from values of coefficients given in Tables D.2 and D.3, | ||
| + | * The design of the tank wall should be by determining the width of the tank wall t and the area of reinforcement required to resist the circumferential tension, shear forces and bending moments determined in the design of slabs, | ||
| + | |||
| + | '''Rectangular Tanks:'''<br> | ||
| + | * In the case of rectangular or polygonal walls, the sides act as two-way slabs, whereby the wall is continued or restrained in the horizontal direction, fixed or hinged at the bottom and hinged or free at the top. The walls thus act as thin plates subjected triangular loading and with boundary conditions varying between full restraint and free edge. | ||
| + | * Analysis for moments and shear forces should be done as that of two-ways slabs considering the walls as individual rectangular slab panels under action | ||
Revision as of 15:36, 30 May 2021
1 APPENDIX D: STRUCTURAL DESIGN OF CONCRETE
Concrete members used in water structures range from walls, slabs, roofs and floors. The design of reinforced concrete should ensure these structures have sufficient resistance to cracking, adequate strength and does not allow leakage. Three methods or approaches are available for structural design of concrete, these are described below:
Working Stress Method:
- Produces uneconomical sections,
- Produces stable sections.
Ultimate Load Method:
- Produces cheaper sections,
- Produces unstable sections.
Limit State Method:
- Produces economical sections,
- Produces stable sections.
For the design of water structures it is recommended to use Limit State Design Method, design of structural members should consider two design limits;
Limit of Collapse Design:
- Take care of safety of structure
- Deals with all types of forces, shear force, bending Moment, torsion moment,
- Design criteria refers to the resistance offered by structure which should not be less than the limit value set in design code,
- The appropriate loading value in the structure is based on loading combination of dead loads, live loads, wind load and earth quake load as provided in BS 8110 code.
Limit of serviceability Design:
- Take care of control, deflection, cracking, abrasion and corrosion,
- The calculated values of deflection shall be less than the permissible values of deflection,
1.1 D.1 Design Requirements and Safety Factors
Design requirements for water structures should be according to BS 8110 but modified for the limits state of cracking to take care of crack width under the effect of applied loads, temperature and moisture content. The details of design requirements and partial safety factors are as per BS 8007 shown below.
1.2 D.2 Criteria for Sizing of Concrete Slabs and Walls
Slabs Resting on Firm Ground:
- Concrete slabs casted to rest directly over firm ground should be designed with nominal percentage of reinforcement provided that it is certain that the ground will carry the load without appreciable subsidence in any part.
- Concrete slabs should be cast in panels with sides not more than 4.5 m with contraction or expansion joints between.
- A screed or concrete layer less than 75mm thick should first be placed on the ground and covered with a sliding layer of bitumen paper or other suitable material to destroy the bond between the screed and floor slab.
- In normal circumstances the screed layer should be of grade not weaker than grade 10, where injurious soils or aggressive water are expected, the screed layer should be of grade not weaker than grade 15 and if necessary a sulphate resisting or other special cement should be used.
Slabs Resting on Support:
- When structures are supported on walls or other similar supports the slabs should be designed as floor in buildings for bending moments due to water load and self-weight.
- When the slab is rigidly connected to the walls (as is generally the case) the bending moments at the junction between the walls and slab should be taken into account in the design of slab together with any direct forces transferred to the slab from the walls or from the slab to the wall due to suspension of the slab from the wall.
- If the walls are non-monolithic with the slab, such as in cases, where movement joints have been provided between the slabs and walls, the slab should be designed only for the vertical loads.
- In continuous T-beams and L-beams with ribs on the side remote from the liquid, the tension in concrete on the liquid side at the face of the supports should not exceed the permissible stresses for controlling cracks in concrete. The width of the slab is determined in usual manner for calculation of the resistance to cracking of T-beam, L-beam sections at supports as given in BS8110 design code.
Circular Tanks with sliding joint at base:
- If the wall of a cylindrical tank has a sliding joint at the base and is free at the top, then when the tank is full, no radial shear or vertical bending occurs, the tank wall will be subjected to pure circumferential tension with a varying
magnitude whereby at bottom there is maximum value and at top there is zero value,
- The design of the tank wall should be done by determining the width of the tank wall t and the area of reinforcement Ast required to resist the circumferential tension only,
- The varying value of circumferential tension per unit height T at depth z below the top and area of steel required to resist circumferential tension and thickness of wall are given by equations below:
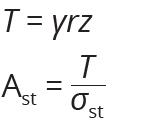
Circular Tank with fixed joint at base:
- If the wall of the Tank is supported at the base such that no radial movement occurs; then the wall will be subjected to radial shear, vertical bending and circumferential tension, the value of circumferential tension is always zero at the bottom of the wall,
- The assumption should be made that some portion of the wall at base acts as cantilever and thus some load at bottom are taken by the cantilever effect. Load in the top portion is taken by the hoop tension. The cantilever effect depends on the height of the wall,
- The bottom part of the tank wall about 13 of the tank height H, or 1 meter from bottom whichever is greater is acted upon by a cantilever moment,
- For walls with free tops and a bottom that is either fixed or hinged values of circumferential tension, vertical moments and radial shear may be calculated from values of coefficients given in Tables D.2 and D.3,
- The design of the tank wall should be by determining the width of the tank wall t and the area of reinforcement required to resist the circumferential tension, shear forces and bending moments determined in the design of slabs,
Rectangular Tanks:
- In the case of rectangular or polygonal walls, the sides act as two-way slabs, whereby the wall is continued or restrained in the horizontal direction, fixed or hinged at the bottom and hinged or free at the top. The walls thus act as thin plates subjected triangular loading and with boundary conditions varying between full restraint and free edge.
- Analysis for moments and shear forces should be done as that of two-ways slabs considering the walls as individual rectangular slab panels under action